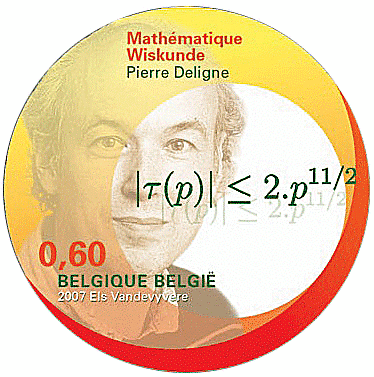
In addition to receiving the 2008 Wolf Prize in Mathematics, Pierre Deligne, Professor Emeritus in the School of Mathematics, has been honored by King Albert II of Belgium, who made him a Vicomte. The Belgian post office has also issued a postage stamp in honor of his achievments in fundamental mathematics.
In assoication with his Vicomte honor, Deligne designed a coat of arms inspired by the song (to the tune of "Twinkle twinkle little star ...”):
Quand trois poules vont aux champs,
La première va devant,
La deuxième suit la première,
La troisième est la dernière,
Quand trois poules vont aux champes,
La premiere va devant.
The song is a tautology, Deligne explained, “and one can view mathematics as being also (long) chains of tautologies.”

At the Institute for Advanced Study, Deligne has brought new insight to deep problems in geometry and number theory. He was a Member (1972–73, 1976–77) and Visitor (1981– 82) in the School of Mathematics and was appointed to the Faculty in 1984. He transferred to Emeritus status as of January.
Born in Brussels on October 3, 1944, Deligne went to the University of Brussels where he was a student of the Belgian algebraist Jacques Tits. While a foreign student at the École Normale Supérieure in Paris, he attended the seminars of Jean-Pierre Serre and of Alexandre Grothendieck. He received his doctorate from the University of Brussels in 1968 and, after a year as a junior scientist at the Fond National de la Recherche Scientifique in Brussels, he joined the Institut des Hautes Etudes Scientifiques, Bures-sur-Yvette.
A member of the Académie Royale de Belgique, the Accademia Nazionale dei Lincei, the American Academy of Arts and Sciences, the National Academy of Sciences, and the Paris Académie des Sciences, Deligne was awarded a Fields Medal in 1978, the Crafoord Prize of the Swedish Academy in 1988 (jointly with Grothendieck), and the Balzan Prize in mathematics in 2004. Other awards include the François Deruyts Prize from the Belgian Royal Academy in 1974, the Henri Poincaré Medal from the Paris Académie des Sciences in 1974, and the A. De Leeuw-Damry-Bourlart Prize in 1975.
When the London Mathematical Society elected Deligne an honorary member in 2003, he was recognized for his monumental contributions to algebraic geometry and for turning Grothendieck’s philosophy of motives from a conjectural program into the driving force behind many of the most subtle areas of current algebraic geometry and arithmetic.
“There are very few mathematicians whose impact on modern mathematics comes close to that of Deligne,” School of Mathematics Professor Peter Sarnak has noted. “Deligne’s research in algebraic geometry and arithmetic geometry have shaped these fields and led him to the solution of a number of long-standing problems, including the Weil Conjectures (which are the analogues of the notorious Riemann Hypothesis for varieties over finite fields) and the celebrated Ramanujan Conjecture in the theory of modular forms.”
Deligne pursues a fundamental understanding of the basic objects of arithmetical algebraic geometry—motives, L-functions, Shimura varieties—and applies the methods of algebraic geometry to trigonometrical sums, linear differential equa- tions and their monodromy, representations of finite groups, and quantization deformation. His research includes work on Hilbert’s 21st problem, Hodge theory, the relations between modular forms, Galois representations and L-series, the theory of moduli, tannakian categories, and configurations of hyperplanes.
In 2005, a conference was held at the Institute in honor of Deligne’s sixty-first birthday. One of the organizers, Hélène Esnault of the Universität Duisburg- Essen, former Member (1993–94) in the School of Mathematics, described Deligne’s work as “having structured the language in which generations of algebraic geometers and arithmeticians think and write.” Deligne has explained the practice of mathematics as akin to searching for a lost key on a street at night— one looks under the light. Esnault observed, “He omitted to mention that to a large extent, he provides the light.”