What Information Might the Topology of Locally Symmetric Spaces Contain?

In 2017–18, I led a special program about analysis and topology on locally symmetric spaces as a Distinguished Visiting Professor in the School of Mathematics. Locally symmetric spaces are the home of the Langlands program—a set of overarching and interconnected conjectures connecting representation theory to number theory, first proposed in 1967 by Robert Langlands, now Professor Emeritus in the School of Mathematics. These spaces have become a crossroads for many different strands of mathematical thought, and the special program placed particular focus on two of these areas:
- Analysis on locally symmetric spaces. The motivation to study this comes both from the Langlands program, and from analytic number theory. The techniques are drawn from representation theory and analysis on manifolds, among other fields.
- Topology of locally symmetric spaces. Here the subject is guided again by a conjecture of Langlands that relates the cohomology of locally symmetric spaces to algebraic varieties. Understanding subtler features of this conjecture was a central theme of the program.
The program was broad and represented several parallel intellectual directions. For coherence, I will focus on just one theme, which suggests that the relationship between the topology of locally symmetric spaces and the arithmetic of algebraic varieties is much richer than expected.
An example of a six-dimensional locally symmetric space
To understand an example of a locally symmetric space, imagine trying to describe the structure of a three-dimensional crystal, i.e., a collection of objects arranged in a repetitive pattern. In nature, there are a bewildering number of variants of crystal structure. The example given here concerns crystals of a simple type, namely, those that arise from stacking copies of a basic cell, as in the picture below.
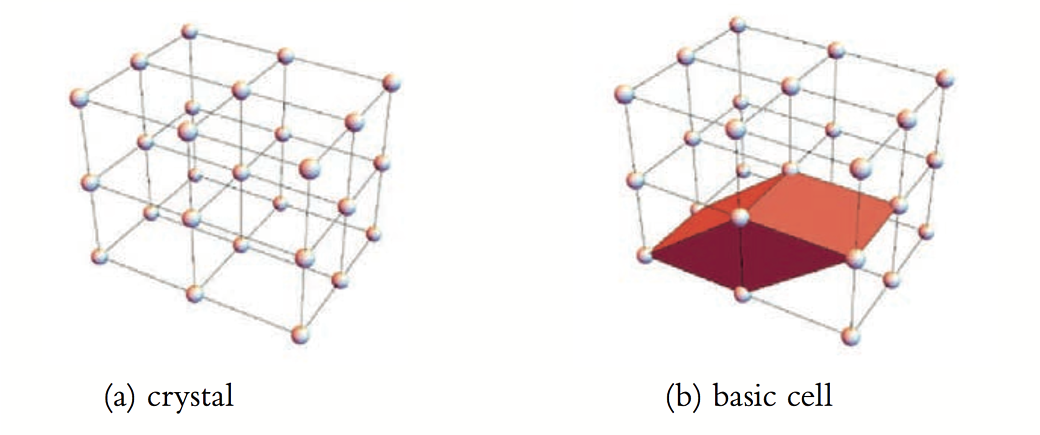
How can we describe this basic cell, which is called a parallelepiped, without the aid of a picture?
We need to give three lengths and three angles. These six numbers completely describe a crystal. If I tell you to construct a crystal whose basic cell has side lengths 2, 2, 3 inches, with the first two sides at an angle of 80 degrees, the last two sides at an angle of 85 degrees, and the first and last at an angle of 75 degrees—there is no remaining ambiguity. You have enough information to go and make a model for this basic cell, and then, by stacking the cell on top of itself, you will reconstruct the crystal.
However, many different basic cells can give rise to the same crystal. See the figure below for an illustration. In the example just given, many other sets of measurements would lead to the same model. This indeterminacy is what makes the situation mathematically complicated.
Now suppose that we make a map of all the possible crystals of this type. Each point on the map describes a specific crystal structure; points on the map are close to one another when their crystal structures are very similar. The resulting map is a basic example of a locally symmetric space. The fact that it requires six coordinates to describe a crystal structure makes this locally symmetric space six-dimensional.

The map is difficult to visualize and work with, because of the indeterminacy mentioned above. One way to work around this would be to fix some way to decide between different basic cells—to settle on a procedure whereby we would only ever use basic cell (a) in the diagram above, for instance. By doing this, we can ensure that each crystal is now represented by a unique collection of six numbers—three lengths and three angles—but the cost is that these numbers must satisfy a complicated collection of constraints.
Mathematically, then, this map of possible crystals looks like a polyhedron, but in six dimensions rather than three. Although hard to picture, we at least know that points on the map represent crystal structures.
This example can be modified in many ways, giving other examples of locally symmetric spaces. For one thing, one can impose specific symmetry axes on the crystal. Or we can work in a different dimension entirely. Each modified situation provides another example of a locally symmetric space, with a corresponding map of all the crystal structures.
Their place in mathematics
Locally symmetric spaces can thus be considered as maps of potential crystal structures. (The mathematical term is lattice, instead of crystal.) They sit at a busy crossroad in mathematics. They provide special but highly interesting example cases in a number of disciplines, including Riemannian geometry, topology, algebraic geometry, and representation theory. But perhaps their richest and deepest interaction is with number theory. I will focus on one small part of this interaction, the relationship between: (i) the topology of locally symmetric spaces, and (ii) the arithmetic of algebraic varieties.
For (i) the topology of locally symmetric spaces, imagine one crystal structure (call it A) slowly morphing until it turns into another one (call it B). (To do this, choose a unit cell for A and imagine slowly altering its lengths and angles until it turns into the unit cell for B.) How do we draw this process on our map? The initial structure and the final structure are both points on the map and the process of morphing corresponds to a path between these two points.
But, as I have emphasized above, there are many choices of a basic cell for crystal A. Suppose we had chosen a different one. We can still slowly alter its lengths and angles so it turns into the basic cell for crystal B. This will give us another path from A to B on our map. These paths are essentially different—there is no intermediate between them. Choosing between them is like deciding to go around an obstacle by going to its left or by going to its right: there is nothing in between.
Topology is concerned with quantifying how many "essentially different" types of paths there can be on our map. It also quantifies higher-dimensional versions of the same question: a path is a one-dimensional structure, but we may also ask how many "essentially different" two-dimensional figures can be drawn on our map, and so on.
Now I will explain (ii) the arithmetic of algebraic varieties, which means the study of polynomial equations with integer coefficients, for example
(1) x3 + 2y3 + 3z3 = 6.
Langlands made the striking prediction that a certain count of solutions to this equation is, in fact, encoded in the topology of locally symmetric spaces.
How do we count solutions? If we permit x, y, z to be arbitrary real numbers, or even whole numbers, the number of solutions may well be infinite. We may avoid this problem by considering the last digits of x, y, and z. How many possibilities are there for these last digits? They can't all be zero—if they were all zero, then the lefthand side of equation (1) would end with a zero, and thus could not equal 6. But (for example) the last digits could all equal 1; or the last digits of x, y, z could be 9, 7, 3 respectively. It turns out that there are exactly one hundred possibilities for the last digits of x, y, z.
This type of counting (based on so-called modular arithmetic) looks rather odd, and it is hard to see why it is a sensible thing to do. But, whatever it is, it is a procedure that belongs to an entirely different world than the geometric objects we described previously. Thus, it is miraculous that (in a way I won't specify) this count of one hundred possibilities shows up, in a very different way, when studying the topology of a certain locally symmetric space.
The special year
I will now explain, somewhat impressionistically, one of the themes of the 2017–18 special year. An algebraic variety is, by definition, just a system of polynomial equations, such as (1) above. A very influential idea of Alexander Grothendieck is that these can be broken up into basic building blocks called motives. The building blocks capture some, although not all, of the information about the variety. For example, the count we did above (how many possibilities for last digits?) can be indicated by the building blocks of the variety. On the other hand, if we ask a more geometric question like "What does the graph of x3 + 2y3 + 3z3 = 6 look like?" it cannot be answered using these building blocks alone. Now, as with many other things, it is much harder to fit the blocks together than it is to break them apart! Speaking a little loosely, the information needed to fit motives together is measured by a very subtle theory that was proposed by former IAS Member Alexander Beilinson and put in its modern form by the late IAS Professor Vladimir Voevodsky—the theory of motivic cohomology.
One keystone of the Langlands program is a relationship between the topology of locally symmetric spaces and the theory of motives. It was this idea that we tried to illustrate in the previous section.
Now, a key goal of the special year was to push this relationship even further. Not only does the topology of locally symmetric spaces contain information about motives (building blocks of algebraic varieties), but it also appears to carry very delicate information about motivic cohomology (which encodes how these blocks fit together). This seems to be a deeper phenomenon, and I find it very surprising. Evidence for it appeared several times over the course of the year—in lectures of Alexander Goncharov, Romyar Sharifi, and myself—although in somewhat different guises each time. The ultimate goal, then, is to make a bridge between the topology of locally symmetric spaces and (a small part of) the world of motivic cohomology. This bridge would carry traffic in both directions: both sides are quite mysterious, but in very different ways! While this bridge must be in some way a part of the Langlands correspondence, much remains to be done in order to understand what it is, where it comes from, and exactly how it fits in with other known phenomena.


