Much of the physical world around us operates on the fundamental principle of “minimization.” It governs everything from the motion of a ball around a roulette wheel to the trajectories of galaxies through spacetime, and is a source of endless fascination for Paul Minter, Veblen Fellow in the Institute’s School of Mathematics. The phenomenon of minimization can be seen throughout nature, where systems seem to “want’’ to take a path that uses the least amount of energy possible to return to an equilibrium state.
Minter explains this with an example: “Imagine a bead threaded through a circular wire hoop which is held in a vertical orientation. If you were to spin the bead around, it would eventually settle at the very bottom of the hoop. This is its point of minimal energy: it needs to expend no energy to ‘keep itself’ here. This bead and hoop system can be described as ‘stable’ because if you slightly perturb the bead, it will always roll back to the same spot.1
But there also exists an “unstable” critical point, which is where the bead balances perfectly on the top of the hoop. This can be considered its point of maximum energy. If you nudge the bead slightly while it is in this position, it will not return to its original location but will instead roll around the hoop. Therefore, despite the top of the hoop being a plausible resting place, this state is described as being “unstable.”
Systems that exhibit a form of stability served as the basis for Minter’s Ph.D. research. In order to explain this, take the bead example a step further: instead of a bead on a circular hoop, now imagine a bead maze that you might have played with as a child, with many undulating wires. The point of minimal energy for the beads on this maze would be the horizontal wooden base at the bottom, but there exist other parts of the maze where the bead would sit in a stable state. For example, when moving the bead along the wire, you might find that it comes to rest in a small trough halfway around the maze, where the wire dips slightly. If you gave the bead a little nudge, it would stay in this position (exhibiting stability). But if you gave it a big push, it would continue to roll along the wire and find its way to the base.

In this case, the minimal point of the whole system (what’s called the “global” minimum) would still be the wooden base. But if you zoomed in and looked at the bead sitting in the trough, considering only its immediate (or “local”) surroundings, this trough would be its most minimal stable point. Minter’s Ph.D. research concerned geometric objects that exhibit these kinds of stable minima—including in far higher dimensions.
When he arrived at the Institute in August 2022, his expertise in this field became key for a project conducted by a pair of his fellow geometric measure theorists: Camillo De Lellis, IBM von Neumann Professor in the School of Mathematics, and Anna Skorobogatova, an IAS visiting graduate student based at Princeton University. The questions which De Lellis and Skorobogatova were tackling pertained to minimizing area, which is closely related to minimizing certain energies. The trio’s collaboration, which forms the subject of this article, has resulted in the publication of a new paper that was highlighted last fall in a report for the National Science Foundation.
Prior to his arrival at IAS, Minter attended a summer conference on the subject of regularity theory at the University of Pisa in Italy. There, he met Skorobogatova and learned of her ongoing work with De Lellis at the Institute. During their initial conversation, he recalls thinking, “Well, the type of situation they need to understand is very similar to the ones I have been thinking about during my Ph.D.!”
The work in which De Lellis and Skorobogatova were engaged can be introduced by means of another example. Instead of playing with a bead maze, now imagine that you are blowing a soap bubble using a simple wand. Why does the bubble that you create take the form of a sphere? Why not an ovoid? Or a cylindrical shape? Or something totally random?
Again, the answer is minimization. Bubbles form a shape that has the least surface area possible within the system’s required constraints. The constraint for the bubble is, of course, the volume of air that has been placed inside. A sphere is the shape that accommodates the required amount of air with the minimal surface area. Thus, bubbles are spherical.

De Lellis and Skorobogatova were investigating what one can say about the geometric structure of more general objects that are governed by minimization principles. To demonstrate the difficulty inherent in providing an answer, imagine that, having admired your first bubble with its minimized surface area, you decide to blow a second. Your two bubbles join in midair, and you notice that they connect in a very specific configuration: the double bubble. This is the shape that minimizes the surface area of the two bubbles combined. But something fundamental has changed about them.
A single bubble is a smooth sphere, but when two bubbles connect, joining points are created, which have “corners.” At these points, described by mathematicians as “singularities,” the bubble ceases to be smooth. Minter, De Lellis, and Skorobogatova are fascinated by what happens when such singularities arise. Their research asks the following: How complicated can singularities be? Can there be lots of singularities? Do the singularities themselves have some sort of structure?
“In this area of research, the ultimate goal would be to provide a full geometric description of a singularity for a variety of different geometric minimization problems. Progress has been made in certain cases, and the problem has been completely resolved in others, but there are many key situations for which we do not yet know the answer,” Minter states. “Full geometric descriptions appear to be very difficult to achieve, but there are softer, related questions that one can ask.”
An important player in the development of this field was Frederick J. Almgren Jr., a frequent Member in the Institute’s School of Mathematics. Almgren’s regularity theorem lays out, in a roughly thousand-page proof, the particular qualities of a singular set of a mass-minimizing surface.2 Prior to his appointment to the Institute Faculty in 2018, De Lellis was well known for producing, alongside his collaborator Emanuele Spadaro, a more accessible version of Almgren’s proof that was more widely understandable to geometric measure theorists. Their work resulted in a concise, up-to-date version of the theory that not only reinforced Almgren’s original claims, but also opened new avenues of inquiry for mathematicians to examine.
The precise area of Almgren’s work that pertains to Minter, De Lellis, and Skorobogatova’s latest endeavor concerned the quantification of singularities. When confronted with geometric objects that have such singularities, Almgren investigated whether he could enumerate how many singularities there were. He was able to show that if one fixes some geometric boundary, and then looks for the surface that spans that boundary with the minimum area (like a soap film stretched within a piece of circular wire), then singularities of that surface always exist in two dimensions fewer than the surface itself.
What does this mean? Let’s first return to the slightly different example of the bubble. In this case, one is not fixing a boundary, but rather fixing the total enclosed volume (i.e., the amount of air inside the bubble), and then finding the minimum surface. That surface is two-dimensional, and, for a double bubble, the singularity which occurs where two bubbles meet takes the form of a one-dimensional line. Thus, the singularity exists in one dimension less than the surface. Almgren’s work was analogous to this, but his starting point was different, since he was interested in soap films with a given boundary rather than bubbles that enclosed a given volume. As a result, his singularities always existed in two dimensions fewer than their associated surface. Unfortunately, this difference means the simplest “surface” that Almgren considered existed in four-dimensions, which is rather hard to visualize!
Almgren’s rule also applies to objects in far higher dimensions than just four. For example, in a 400-dimensional object in 1,000-dimensional space, the singularities could be 398-dimensional. “Understanding the number of possible singularities through their dimension is a useful way of starting to describing their structure,” Minter continues. “But it remains something of a qualitative statement. It cannot, for example, tell you anything about the local structure of singularities and it does not help to classify them.”
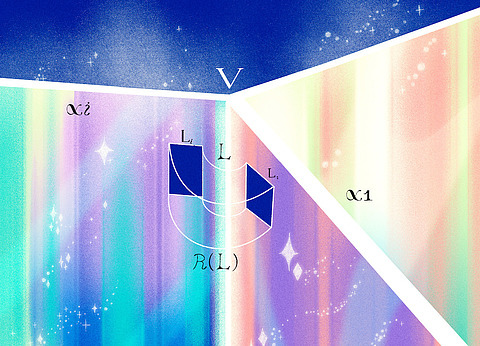
To study singularities in a more precise way, Minter, De Lellis, and Skorobogatova investigated their local geometric structure. In simple terms, they put the singularity under a microscope. In the case of the double bubble singularity, zooming in on the connection point between the two bubbles showed that the bubbles met along a connection point in the form of a geometric structure, namely a line. Continuing to zoom in, they observed that the surfaces began to look like three flat planes that ultimately intersect at the singularity, forming a type of Y-shape. The technical name for these intersecting planes is a “tangent cone.”
In the case of bubbles, only two different types of singularities are observed: Y-type singularities in double bubbles and another type which looks like a tetrahedron, seen in triple bubble clusters. But in other objects, especially in higher dimensions, singularities can look very different. Minter and his collaborators were able to show that these singularities in high dimensions are not arbitrary. Instead, they have a certain geometric structure. Moreover, they were able to prove that for the vast majority of singularities the tangent cone is unique. In other words, they demonstrated that when you put a typical singularity under a microscope, you see a fixed shape (analogous to the Y-shape in the double bubble) as you zoom in.3 To summarize, Minter, De Lellis, and Skorobogatova’s work has enhanced our understanding of what kind of structures form minimal surfaces.
Collaboration was key in the success of the project. “Working collaboratively made it much simpler because we had people with expertise on each side of things,” Minter outlines. But there was no single Eureka! moment where everything came together. Instead, Minter describes the “nervous anticipation” that the trio felt as their work progressed: “In any project, there come points where the math seems to be working, and then there are some horrendous technicalities that you have to sit down and work through. I think at the end, we breathed a sigh and thought, ‘Phew, everything is going to work out.’”
Minter, De Lellis, and Skorobogatova are continuing their collaborative work with a follow-up project, which is related to objects which minimize area “mod p.” Mod p numbers can be described as remainders which result when natural numbers (i.e., the numbers that you use for counting, e.g., 1, 2, 3, all the way to infinity) are divided by p (a prime number). The trio’s research into area minimizing objects has provided a machinery which might be applicable to this related problem. “Now,” Minter says, “we are trying to adapt our machinery to this new problem. And currently, things are looking promising.”
In the words of Swiss mathematician Leonhard Euler over 300 years ago, “Nothing at all takes place in the universe in which some rule of maximum or minimum does not appear,” meaning that the principle of minimization will provide scholars with intriguing questions for many more years to come.
Paul Minter is a Veblen Fellow in the Institute’s School of Mathematics. He came to IAS as a Veblen Research Instructor (2022–23), after completing his Ph.D. at the University of Cambridge. He was awarded a Clay Research Fellowship in 2023. To date, his focus has been at the interface between geometry and mathematical analysis, trying to understand special geometric structures which arise and their relation to geometry and topology.
[1] Stability is important: in the real world, one would not expect for unstable states to survive for very long. This is because a small influx of energy, which would be commonplace in a real-world setting, will cause an unstable system to be disrupted. Therefore, one expects the majority of systems to be stable.
[2] Almgren’s initial work on the regularity theorem was completed in 1983. This was followed by a posthumous publication in the year 2000.
[3] These conclusions were independently reached at the same time by another pair of scholars, Brian Krummel (University of Melbourne) and 2019 Member in the School of Mathematics Neshan Wickramasekera (University of Cambridge), using different methods.